Marginal Cost and Average Total Cost (transcript)

Marginal cost and average total cost can be derived from the short-run total cost subject to the law of diminishing returns.

From production to cost

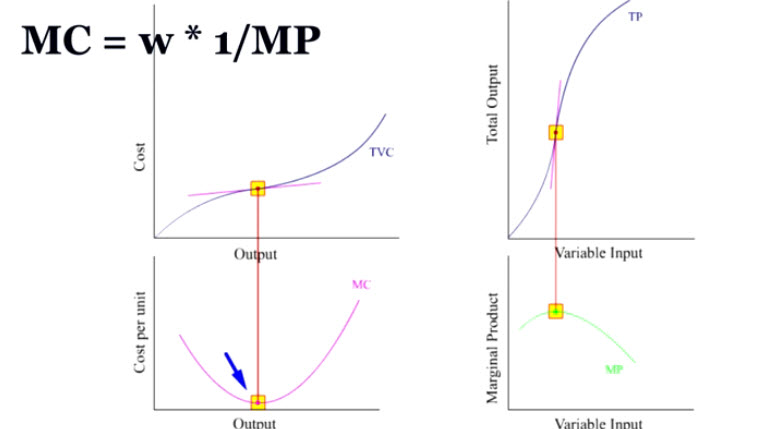
In the short run where some fixed inputs are combined with variable inputs, the production function is an S-shaped upward sloping curve (TP). This total product curve starts with a segment with increasing slope followed by a segment with decreasing slope reflecting the law of diminishing returns.

From this TP curve, we can derive the total variable cost curve.

In the TP curve, output is a function of variable input. By reversing the horizontal and vertical axis, we get TVI (total variable input).

The new (flipped) curve shows variable input as a function of output.

If we multiply TVI by a constant unit cost of the variable input, we get TVC (total variable cost). Here we assume the constant unit cost is $1, so the vertical axis retains the same scale and is simply relabeled as cost.

The increasing-slope segment of TP...

is mirrored by the decreasing-slope segment of TVC.

The decreasing-slope segment of TP...

is mirrored by the increasing-slope segment of TVC.

The inflection point of TP...

is mirrored by the inflection point of TVC.

Generating MC from TVC

Just as we can derive the marginal product curve(MP) from the TP curve by measuring the slope of the tangents to the TP curve, we can derive the marginal cost (MC) curve from TVC.

While MP is the additional output from additional variable inputs, MC is the additional cost from additional output.

Given the inverted S-shape of TVC, MC first decreases...

until MC reaches its minimum at the inflection point.

Then, MC increases after the inflection point.

Thus, the inverted S-shaped TVC generates a U-shaped MC.

From MP to MC

Since TVC is a mirrored image of TP, there is a one-to-one correspondence between segments of the two curves.

When MP is increasing, MC is decreasing.

When MP is decreasing, MC is increasing.

So when MP reaches its maximum, MC reaches its minimum.

These correspondences occur because

1. TVC is a mirrored image of TP

2. MC=w*1/MP where w = constant unit cost of variable input

Generating ATC from TC

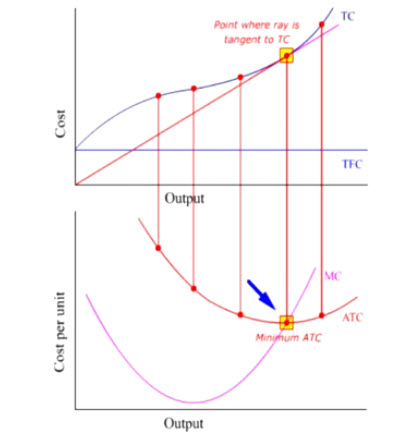
When the cost of fixed inputs is added, TVC is simply shifted up by the same amount over the whole range of output.

The result is therefore TC (total cost) which is the sum of TFC (total fixed cost) and TVC (total variable cost)

For example, at point 'A': TC = a + b where a = TVC, b = TFC

Since the slope of TVC stays the same, shifting the TVC up by the fixed cost does not affect the value of MC.

When TC is divided by a given level of total output, we get ATC (average total cost)

At Q1, ATC = TC1/Q1.

Since TC1/Q1 also measures the slope of the ray from the origin to TC, the ray slope provides a visual indication of the numerical value of ATC.

The numerical value of ATC is plotted on the graph below.

Given the S-shape of TC, ATC first decreases until it reaches the point on TC where the ray from the origin is tangent to TC.

Afterwards, the slope of rays from the origin to TC starts to increase giving ATC a U-shape.

Observations

1. ATC reaches its minimum when the ray from the origin is tangent to TC.

Since the slope of this tangency measures both MC and ATC, MC therefore intersects ATC at ATC's minimum point

2. ATC has a symmetric U-shape because two points on opposite sides of the minimum ATC point on TC have equal slopes.

3. When ATC is decreasing, MC is always below ATC. MC could be falling or rising.

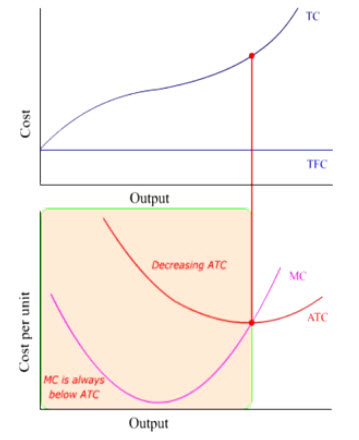
4. When ATC is increasing, MC is always above ATC and rising.

Why MC and ATC?

Why do we need MC and ATC when we have TC?

We need ATC because it is convenient to compare price with ATC to get an idea whether we are at least breaking even. And we need MC because pricing should be based on MC not on ATC. To maximize profit, we need to know that unit price is not only higher than ATC (thus covering all costs), but also that the additional cost of the marginal unit (MC) is not higher than the unit price.